
Two irrational integers added together are still irrational, but only sometimes. The addition of two rational numbers results in the production of a third rational number. Since integers are generally closed under addition and multiplication, adding two rational numbers is the same as adding two fractions of the same form, which will result in another fraction of the same form since integers have been closed under addition. A rational number may be stated as a fraction having integer values in both the numerator and the denominator, according to the meaning of the term (denominator not zero). The addition of two rationales also results in a rational number. Non-perfect square roots, for example, will always yield an irrational value. This implies that irrational numbers can’t be stated as the ratio of two other integers in any way.

Most irrational numbers are regarded as real numbers in mathematics, which is not rational. Do Irrational Numbers Represent Real Numbers? Calculus and other mathematical domains that use these irrational numbers are used a lot in real life. Engineering revolves around designing things for real life, and several things like Signal Processing, Force Calculations, speedometers, etc., use irrational numbers. These components may not directly be used, but are used extensively in Engineering.
Euler’s Number e – Used extensively in Logarithms and Algebra. Several Integrals use it, and it is also used in Polar Coordinates Pi (π) – Circles make no sense without π. The ratios are used in various height and distance measurements and also in several calculations in physics. 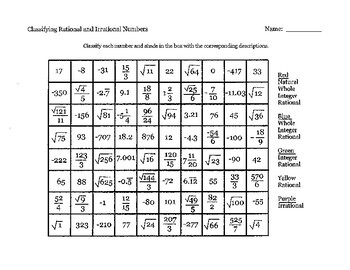
Trigonometric Ratios need irrational numbers.
Obviously, you may not be able to visualize it, but several components use irrational numbers. Irrational numbers are used in various components of life. What Applications Do Irrational Numbers Have In Everyday Life?
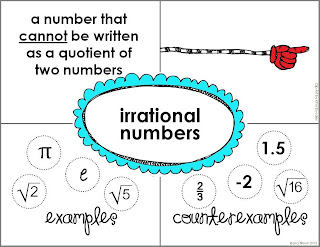
In contrast to the set containing rational numbers, the set containing irrational numbers does not become closed when the process of multiplication is applied to it. There is no guarantee that the (LCM) of any pair of irrational numbers even exists. As a result, we can conclude that xy is an irrational product. Let us assume that x is irrational, but if xy=z, then x = z/y is rational. Any irrational number multiplied by a nonzero rational number yields another irrational number. For illustration, suppose x is irrational, and y is rational, and that the sum of the two, x + y, is also irrational. Irrational numbers can be created by adding them to rational ones. Irrational numbers have the following characteristics as a result of their nature: The calculated value of pi is 3.141592653589………….Īn illustration of pi, which is irrational, is shown below.Īn illustration of Euler’s number e, which is irrational, is shown below.įigure 3 – Irrational number Irrational Numbers’ Inherent CharacteristicsĪs a result of the fact that irrational numbers are subsets of real numbers, irrational numbers are subject to all of the characteristics that are associated with the real number system. In addition, 22/7 is indeed a rational number, whereas pi is indeed an irrational number hence, the two quantities cannot be comparable. Pi is considered to be an irrational number because it does not terminate or repeat in decimal form. In contrast, the term “rational number” refers to any number that can be expressed as the fraction p/q, provided that both p and q are integers and that q is not equal to zero. Irrational expressions include things like 2 and 3, amongst others. These real numbers are known as being irrational. Irrational numbers are real numbers that cannot be stated in the form of p/q, where both p and q are integers and q is less than zero. How Can You Tell Whether a Number Is Irrational? What Sort of Numbers Fall Within this Category? We cannot represent any irrational number as a ratio, such as p/q, where p and q are integers and q is less than zero. For example, -2 is indeed an irrational number because it can’t be represented as a ratio of two integers. 
Irrational numbers are real numbers that cannot be represented like a ratio of integers. The following figure shows the irrational number.įigure 1 – Hypotenuse showing the irrational number What Constitutes Irrational Numbers? Irrational numbers are typically written as R\Q, where the backslash signifies “set minus.” It is also possible to write the difference between the real numbers and the rational numbers as R – Q. It is inconsistent with rational numbers. An irrational number cannot be written as just a ratio, such as p/q, where p and q are positive integers and q is greater than zero. Irrational numbers are those that cannot be expressed as fractions.








 0 kommentar(er)
0 kommentar(er)
